Head Bernoulli Equation in Process Engineering

Head Bernoulli is a term that refers to the total energy of a fluid at a given point in a steady flow system. It consists of three components: elevation head, velocity head, and pressure head. These components represent the potential, kinetic, and pressure-related energies of the fluid, respectively.

Elevation head is the energy due to the height of the fluid above a reference level. Velocity head is the energy due to the speed of the fluid. Pressure head is the energy due to the pressure exerted by the fluid.

According to Bernoulli’s principle, the head Bernoulli of a fluid is constant along a streamline, as long as there is no friction, heat transfer, or work done on or by the fluid. This means that if one component of the head Bernoulli increases, one or more of the others will decrease to compensate, and vice versa.

For example, if a fluid flows through a pipe with varying cross-sectional area, the velocity head will increase as the area decreases, and the pressure head will decrease as the area decreases. The elevation head will remain constant if the pipe is horizontal. Therefore, the head Bernoulli will be the same at any point in the pipe.

However, in real systems, there are always some energy losses due to friction, turbulence, or other factors. These losses are called head losses, and they reduce the head Bernoulli of the fluid. To account for these losses, the modified Bernoulli equation includes a term for the head loss.

Similarly, if there is a pump or a turbine in the system, there will be some work done on or by the fluid, which will change the head Bernoulli of the fluid. To account for this work, the modified Bernoulli equation includes a term for the pump work or the turbine work.

Understanding the Head Bernoulli Equation

The Head Bernoulli Equation, derived from the classic Bernoulli equation, is a representation of energy conservation in fluid flow. It states that the total mechanical energy per unit weight of fluid along a streamline remains constant, neglecting losses due to friction, heat transfer, and other dissipative processes. Mathematically, the equation can be expressed as:

$\frac{P}{\rho g} + z + \frac{V^2}{2g} = H$

Where:

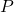$P$ = Pressure of the fluid
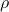$\rho$ = Density of the fluid
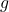$g$ = Acceleration due to gravity
$z$ = Elevation of the fluid above a reference point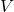
$V$ = Velocity of the fluid
$H$ = Total mechanical energy per unit weight (also known as head)

This equation essentially represents the conservation of mechanical energy, where the sum of pressure head, elevation head, and velocity head remains constant along a streamline in steady, incompressible flow.

Practical Example: Pumping System Design

Let’s consider a scenario where a process engineer is tasked with designing a pumping system to transport water from a reservoir to a storage tank located at a higher elevation. The engineer needs to determine the required pump head to achieve the desired flow rate.

Given:

Reservoir water level: 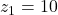$z_1 = 10$ meters
Storage tank elevation: 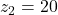$z_2 = 20$ meters
Desired flow rate: 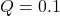$Q = 0.1$ m³/s
Density of water: 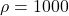$\rho = 1000$ kg/m³
Acceleration due to gravity: 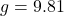$g = 9.81$ m/s²
Negligible losses

Using the Head Bernoulli Equation, we can calculate the required pump head ( $H$ ) as follows:

$H = z_2 - z_1 + \frac{V^2}{2g}$

Given that the flow rate $Q = \frac{A \cdot V}{A}$ , where $A$ is the cross-sectional area of the pipe, and $V$ is the velocity of the fluid. We can rearrange to solve for $V$ :