Understanding Bernoulli's Equation with Friction in Process Engineering

The Bernoulli equation is a way of describing how the energy of a fluid changes along its path. It says that the sum of the pressure energy, the kinetic energy, and the potential energy of a fluid is constant, as long as the fluid is incompressible, frictionless, and steady.

However, in reality, most fluids experience some friction, which means that some of their energy is lost as heat. This reduces the pressure and the velocity of the fluid along its path. To account for this, we can add a term to the Bernoulli equation that represents the energy loss due to friction. This term is usually proportional to the square of the fluid velocity and the length of the path.

To explain this without formula, let’s consider an example of water flowing through a pipe that has a constriction. When the water enters the narrow part of the pipe, it speeds up, because the same amount of water has to pass through a smaller area. This increases the kinetic energy of the water, but decreases its pressure energy, according to the Bernoulli equation. However, because of friction, some of the kinetic energy is also lost as heat, which further reduces the pressure energy. This means that the pressure at the narrow part of the pipe is lower than the pressure at the wider part of the pipe, even after accounting for the change in velocity.

The Basics of the Bernoulli Equation with Friction:

The Bernoulli equation describes the conservation of energy along a streamline in a fluid flow. When considering frictional effects, the equation takes the following form:

$P_1 + \frac{1}{2}\rho v_1^2 + \rho gh_1 = P_2 + \frac{1}{2}\rho v_2^2 + \rho gh_2 - \Delta P_f$

Where:

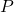$P$ represents pressure,
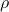$\rho$ is the fluid density,
$v$ denotes velocity,
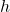$h$ signifies height above a reference point,
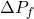$\Delta P_f$ represents the pressure loss due to friction.

Example Scenario:

Let’s consider a horizontal pipe with water flowing through it. The diameter of the pipe is 0.1 meters, and the flow rate is 0.05 m³/s. The pressure at point 1 is 200 kPa, and at point 2, it is 150 kPa. The height difference between the two points is negligible. We want to calculate the pressure loss due to friction.

Calculation and Results:

To calculate the pressure loss due to friction, we first need to determine the velocity at points 1 and 2. We can use the continuity equation to find the velocity:

$Q = A_1 \times v_1 = A_2 \times v_2$

Where $Q$ is the flow rate and $A$ is the cross-sectional area.

Given $Q = 0.05$ m³/s and $A = \pi \times (0.1/2)^2$ , we can find $v_1$ and $v_2$ :

$v_1 = \frac{Q}{A_1} = \frac{0.05}{\pi \times (0.1/2)^2} \approx 6.37 \, \text{m/s}$

$v_2 = \frac{Q}{A_2} = \frac{0.05}{\pi \times (0.1/2)^2} \approx 6.37 \, \text{m/s}$

Next, we can use the Bernoulli equation with friction to find the pressure loss:

$P_1 + \frac{1}{2}\rho v_1^2 = P_2 + \frac{1}{2}\rho v_2^2 + \Delta P_f$

$200 \, \text{kPa} + \frac{1}{2} \times 1000 \, \text{kg/m³} \times (6.37 \, \text{m/s})^2 = 150 \, \text{kPa} + \frac{1}{2} \times 1000 \, \text{kg/m³} \times (6.37 \, \text{m/s})^2 + \Delta P_f$